Answers
Coffee Break
Brain Challenge
Stuck for an answer to one of our Brain Challenges? They’re all here…
Week 229 (17 December 2021)
There are 10 presents under the Artemis Clarke Christmas tree.
5 of the presents are wrapped in gold paper.
3 of the presents are wrapped in silver paper.
2 of the presents are wrapped in red paper.
Kate opens 2 of the presents from under the Christmas tree.
What is the probability that both presents are wrapped in the same coloured paper?
Answer:
Prob of 2 gold presents = 5/10 x 4/9 = 20/90
Prob of 2 silver presents = 3/10 x 2/9 = 6/90
Prob of 2 red presents = 2/10 x 1/9 = 2/90
So total probability = 20/90 + 6/90 + 2/90 = 28/90 = 14/45
Week 228 (10 December 2021)
Simon is singing Christmas carols in church with his choir.
There are 60 people in the choir.
Half of the people in the choir are women.
The number of women in the choir is 3 times the number of men in the choir.
The rest of the people in the choir are children.
The number of children : the number of men in the choir = n : 1
Work out the value of n.
Answer:
W = 60/2
3M = W
Therefore:
3M = 60/2
M = 60/6 =10
C = 60 –3M – M = 20
So Children: Men = 2:1
Therefore n = 2
Week 227 (3 December 2021)
Ruby is making biscuits for a Christmas party.
Ruby needs 50g of sugar to make 15 biscuits.
She also needs three times as much flour as sugar and two times as much butter as sugar to make the 15 biscuits.
Ruby decides that for the party she is going to make a larger batch of biscuits.
Work out the amount of flour that she needs to make 60 biscuits.
Answer:
For 60 biscuits Ruby will need 60/15 x 50g = 200g of sugar
She will therefore need 3 x this for the flour = 3 x 200g = 600g
Week 226 (26 November 2021)
During her lunch break Carol walks to the shop to pick up a meal deal.
There are 8 different sandwiches and 4 different drinks.
Two of the sandwiches have cheese in them and three of the drinks are fizzy. Carol picks a meal deal at random.
Work out the probability that the sandwich has cheese in it and the drink is fizzy. Give your answer as a fraction.
Answer:
2/8 X 3/4 = 6/32 = 3/16
3/16
Week 225 (19 November 2021)
Ellie has nearly finished her maths homework but is struggling with the last question on sequences.
What comes next in the following sequence:
136 101 521 283 645 556 ?
Can you help Ellie find the next number in the sequence?
Answer:
The sequence is actually using triangular numbers (1, 1+2 = 3, 3+3 = 6, 6+4 = 10 etc) which have been grouped into threes.
So 55 +11 = 66
And 66 + 12 = 78
The first 6 forms part of the 556, so the next term is 678
Week 224 (12 November 2021)
Emily has been working hard on her hockey skills over the past few years and in recognition of her hard work she was selected to play in a higher league.
There are 16 hockey teams in the higher league.
Each team has played two matches against each of the other teams.
Work out the total number of matches played.
Answer:
For team 1 to play the other 15 teams once, they must play 15 games. For team 2 to play the other 15 teams once it also must play 15 games, but the game against team 1 is already included in the count for team 1. So there must be 14 more games. Similarly for team 3 we need 13 more games.
So for all 16 teams to play each other once, we need 15 + 14 + 13 + … + 3 + 2 + 1 = 15(16)/2 = 120 games.
For all teams to play each other twice, we need another 120 games.
We need 2(120) = 240 games.
Week 223 (5 November 2021)
Sarah and Matt are at a fireworks display with their friends.
They buy 3 toffee apples and 2 hot dogs which costs a total of £7.80.
Later in the evening they buy a further 5 toffee apples and 4 hot dogs which costs a total of £14.20.
Work out the cost of one toffee apple and the cost of one hot dog.
Answer:
3 TA + 2 HD = 7.80
6 TA + 4 HD = 15.60
4 HD = 15.60 – 6 TA
Substituting into:
5 TA + 4 HD = 14.20
5 TA + 15.60 – 6 TA = 14.20
TA = 15.60 – 14.20 = 1.40
Therefore:
3 x 1.40 + 2 HD = 7.80
2 HD = 3.60
HD = 1.80
So:
Toffee apple = £1.40
Hot dog = £1.80
Week 222 (29 October 2021)
y is inversely proportional to d²
When d = 10, y = 4
d is directly proportional to x²
When x = 2, d = 24
Find a formula for y in terms of x.
Give your answer in its simplest form
Answer:
y= K/ d²
where K is a constant
K = y * d²
So when d = 10 and y = 4:
K = 4 * 100 = 400
Substituting in K:
Therefore d2 = 400/ y
y = 400/ d²
Also:
d = C * x²
d/ x² = C
24/ 4 = C
C = 6
So:
d= 6 x²
Substituting back into:
y = 400/ d²
y = 400/ (36 x⁴)
y = 100/ (9 x⁴)
Week 221 (22 October 2021)
A local charity is trying to raise money and decides to host a fun day.
Ava is in charge of the game of chance.
The spinning wheel is marked with the numbers 1 to 10.
A player spins once and wins £2.00 if the spinner lands on 6.
Fin plays the game exactly twice.
Work out the probability that Fin wins £4.00.
Answer:
Probability of winning = 1/10
If win twice then probability = 1/10 x 1/10 = 1/100
Week 220 (15 October 2021)
Josh and Rosie are renovating their house and the cost of building a single storey house extension is in the ratio:
Labour : Materials : Professional Fees = 7 : 5 : 1
The cost of materials is £6400.00 more than the amount paid in professional fees.
Work out Josh and Rosie’s total cost of building the single storey house extension.
Answer :
L:M:P
M = 5P
P + 6400 = M
Therefore
(P + 6400) = 5P
4P = 6400
P = 1600
Therefore total cost = 13 x 1600 = £20,800
Week 219 (8 October 2021)
Jenny inherits some money and decides to invest £12000.00 in an account paying compound interest for 2 years.
In the first year the rate of interest is x%
At the end of the first year the value of Jenny’s investment is £12336.00
In the second year the rate of interest is x/2%
What is the value of Jenny’s investment at the end of 2 years?
Answer:
Rate of interest in first year = (12336 -12000)/ 12000 x 100% = 2.8%
Rate in 2nd year = 2.8/ 2 = 1.4%
So investment at end of 2nd year = 1.014 x 12336 = £12,508.70
Week 218 (1 October 2021)
A factory makes 450 pies every day.
The pies are either chicken pies or steak and kidney pies.
Each day Chris takes a random sample of 15 pies to check the quality.
The proportion of the pies in his sample that are chicken is the same as the proportion of the pies made that day that are chicken.
On Monday Chris calculated that he needed exactly 4 chicken pies in his sample.
Work out the total number of chicken pies that were made on Monday.
Answer:
4/15 x 450 = 120
Week 217 (24 September 2021)
In a survey 50 people were asked if they liked tea, coffee or hot chocolate.
Every person liked at least one of the drinks.
17 people liked all the drinks.
31 people liked hot chocolate.
34 people liked tea.
21 people liked tea and coffee.
7 people liked tea and hot chocolate but not coffee.
2 people liked coffee and hot chocolate but not tea.
Two of the 50 people are chosen at random, what is the probability that they both like coffee?
Answer:
The easiest way is to draw a Venn Diagram:
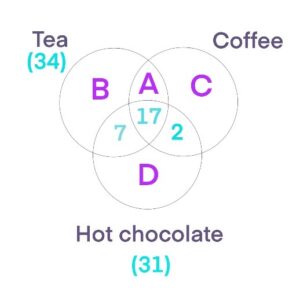
21 like tea and coffee (which can include people that like hot chocolate), but as we know 17 like all three drinks, only 4 people like just tea and coffee (ie 21 – 17), which is A in the Venn Diagram.
People who only like hot chocolate (D) = 31 – 7 – 2 – 17 = 5
So people who only like coffee (C) = 50 – 34 (ie tea drinkers) – 2 – 5 (ie D) = 9
Therefore the total coffee drinkers = C + A + 17 + 2 = 32
Therefore probability that they both like coffee = 32/50 x 31/49 = 992/2450 = 496/1225
Week 216 (17 September 2021)
In 2017, Jerry bought a small house as an investment.
In 2018, Jerry decided to cash in his investment and sold the house to Amelia.
Jerry made a profit of 20% (as a % of the cost).
In 2020, Amelia decided to sell the house. Amelia sold the house for £162,000.
Amelia made a loss of 10% (as a % of the cost).
Work out how much Jerry initially paid for the house in 2017.
Answer:
Firstly we need to work out how much Amelia paid for the house:
Profit/ loss = (Selling price – cost)/ cost x 100%
Therefore
-0.1 x cost (A) = selling price – cost (A)
0.9 cost (A) = selling price
Cost = 162,000 / 0.9 = 180,000
This is therefore the sales price for Jerry, so:
0.2 x cost (J) = 180,000 – cost (J)
Cost (J) = 180,000/ 1.2 = 150,000
So Jerry paid £150,000
Week 215 (10 September 2021)
A cuboid container is going to be filled with water.
The dimensions are length 120cm, width 70cm and height 50cm.
Water will flow from a hose into the container at a rate of 2 litres per second.
How long in minutes will it take for the container to completely fill?
Answer:
Volume = 50 x 70 x 120 = 420,000 cm^3
To convert to litres, divide by 1000
So, volume in litres = 420,000 / 1000 = 420 litres
420 / 2 = 210 seconds
Divide by 60 to get minutes:
So time taken = 210/60 = 3.5 minutes.
Week 214 (3 September 2021)
Jake buys 5 kg of sweets to sell
he pays £10 for the sweets.
Jake puts all the sweets into bags
he puts 250 g of sweets into each bag
he sells each bag of sweets for 65p
Jake sells all the bags of sweets
Work out his percentage profit.
Answer :
Total number of bags of sweets = 5000/250 = 20 bags
Total revenue = 0.65 x 20 = £13
Therefore, percentage profit = 3/10 x 100% =30%
Week 213 (27 August 2021)
A force of 70 newtons acts on an area of 20cm^2
The force is increased by 10 newtons.
The areas increased by 10cm^2
Helen says
“the pressure decreases by less than 20%”
Is Helen correct?
You must show how you get your answer
Answer:
Pressure = Force/ Area
P1 = 70/20 = 3.5
P2 = (70+10)/(20 +10) = 2.667
% decrease = P2-P1/P1 = (3.5-2.667)/ 3.5 x 100% = 23.81%
Which is more than 20%, so NO
Week 212 (20 August 2021)
Two packs of tea bags are available at the local corner shop.
Pack A contains 240 tea bags for £4.00 plus 20% extra free.
Pack B contains 240 tea bags and has a discount of 15% off the normal price of £4.00.
Which pack offers the best value for money?
Answer:
Pack A
240 bags plus 20% = 288 bags
So cost per bag = 400p/288 = 1.39p per bag
Pack B
240 bags cost £3.40 (0.85 * £4)
So cost per bag = 340/240 = 1.42p per bag
So Pack A offers better value
Week 211 (13 August 2021)
To take his mind off his upcoming GCSE results, Austin was doing a jigsaw puzzle.
The puzzle has 700 pieces.
What is the smallest possible number of edge (including corner) pieces that it could have?.
Answer:
Perimeter is least when the sides are 28 x 25
Number of edge pieces are then 2 x 28 = 56 along the longest sides
But we now have only 23 along the shortest sides making 2 x 23 = 46
Hence total is 56 + 46 = 102
Week 210 (6 August 2021)
225g of flour are needed to make 9 cakes.
Julie wants to make 20 of these cakes. She has 475g of flour.
Does Julie have enough flour to make 20 cakes?
Answer:
Amount of flour required per cake = 225/9 = 25g
Therefore to make 20 cakes she would need = 25 x 20 = 500g
So NO, she is 25g short and can only make 19 cakes
Week 209 (30 July 2021)
There are 3 faulty floodlights in a sports stadium.
Floodlight A flashes every 20 seconds.
Floodlight B flashes every 45 seconds.
Floodlight C flashes every 120 seconds.
The three lights start flashing at the same time.
How many times in one hour will the three lights flash at the same time?
Answer:
Need to work out the lowest common multiple, which is 360.
Number of seconds in an hour = 60 x 60 = 3,600.
Therefore the number of times they flash at the same time = 3600/360 = 10
However if they flash at time zero as well, then it would be 11 (we’ve marked both as correct 
Week 208 (23 July 2021)
There are 11 pens left in the office stationery cupboard.
8 of the pens are black and 3 of the pens are blue.
Two pens are taken out of the cupboard at random without replacement.
Work out the probability that the two pens are the same colour.
Answer:
8/11 x 7/10 + 3/ 11 x 2/10 =56/110 + 6/110 = 62/110 = 31/55 or 56.36%
Week 207 (16 July 2021)
66 people went on a day trip.
Each person did only one activity on the trip.
The options were to go skating, visit an art gallery or go bowling.
43 of the people were female.
4 of the 10 people who went skating were male.
20 of the people went to the art gallery.
10 males went bowling.
Work out the number of females who went to the art gallery.
Answer:
There were 23 men in total (66 -43).
9 men must have gone to the art gallery (ie 23 – 4 – 10).
Therefore 11 women must have gone to the art gallery (20 – 9).
So the answer is 11
Week 206 (9 July 21)
William is going to buy tickets for his family to watch a football match.
4 adult tickets cost £15 each and 2 child tickets cost £10 each.
A 10% booking fee is added to the ticket price.
3% is then added for paying by credit card.
Work out the total charge for these tickets when paying by credit card.
Answer:
Cost of tickets = 4 x 15 + 2 x 10 = £80
Booking fee = 0.1 x 80 = £8
Credit card fee = £88 x 1.03 = £90.64
Week 205 (2 July 2021)
Josh is taking his scout group on a camping trip. He buys tins of soup and bottles of water.
He needs to buy the same number of tins as bottles and take them in their unopened packs.
Tins of soup are sold in packs of 12 and bottles of water are sold in packs of 15.
What is the smallest number of packs of each that Josh can buy?
Answer:
We need to find the lowest common multiple of 12 and 15. This is 60.
So 60/12 = 5
60/15 = 4
Packs of soup = 5
Packs of water = 4
Week 204 (25 June 2021)
A football team is going to play a match on Saturday and on Sunday.
The probability that the team will win on Saturday is 0.45
If they win on Saturday, the probability that they will win on Sunday is 0.67
If they do not win on Saturday, the probability that they will win on Sunday is 0.35
Find the probability that the team will win exactly one of the two matches
Answer:
If win then lose, probability = 0.45 x 0.33 = 0.1485
If lose then win, probability = 0.55 x 0.35 = 0.1925
Total probability = 0.1485 + 0.1925 = 0.341
Week 203 (18 June 2021)
There is a patch of lily pads on a lake. Everyday, the patch doubles in size
If it takes 48 days for the patch to cover the entire lake, how long would it take for the patch to cover half of the lake.
Answer:
47 (as it will double in size the next day)
Week 202 (11 June 2021)
A sushi restaurant buys 20 fish for £10 each. The owner knows that 50% of the fish will go bad before being served. Each fish creates 10 servings. What price must they charge per serving in order to make 100% profit on their initial investment?
Answer:
Cost = £200 (20 x 10)
10 fish bad
100 servings (10 x 10)
100% profit = £400
400/100 = £4 per serving
Week 201 (4 June 2021)
Emma is tiling her bathroom. She needs 100 tiles to complete her bathroom. At the shop the tiles are sold in large packs and small packs. The cost of a small pack (34 tiles) is £14, the cost of a large pack (40 tiles) is £18. There is an offer of a 25% reduction when you buy three or more large packs. Work out the cheapest cost for Emma to buy the amount of tiles she needs.
Answer:
Small packs = 3 X 14 = £42
Large packs = 3 x 18 x 0.75 = £40.50
So buying the large packs would be the cheapest
Week 200 (28 May 2021)
At the local school there are 50 students.
15 play tennis, 20 play cricket and 20 do athletics. 3 play tennis and cricket, 6 play cricket and do athletics, and 5 play tennis as well as doing athletics.
7 play no games at all.
How many play cricket, tennis and do athletics?
Answer:
Let w = tennis + athletics but not cricket
Let x = tennis + cricket, but not athletics
Let z = cricket + athletics, but not tennis
Let y = cricket + athletics + tennis
Therefore:
x + y = 3; so x = 3 – y
y + z = 6; so z = 6 – y
w + y = 5; so w = 5 – y
50 = 7 + 20 + 20 – x – y – z + 15 – y – w
So:
50 = 62 – x – 2y – z – w
x + 2y + z + w = 12
Substituting in:
3 – y + 2y + 6 – y + 5 – y = 12
14 – y = 12
Y = 2
Week 199 (21 May 2021)
In a village the number of houses and the number of flats are in the ratio 7:4
The number of flats and the number of bungalows are in the ratio 8:5
There are 50 bungalows in the village
How many houses are there in the village?
Answer:
Flats: Bungalows = 8:5 = 80:50
Houses: Flats 7:4 = 140:80
Therefore there are 140 houses
Week 198 (14 May 2021)
The number of voters visiting a polling station in 2020 was 438048.
This was an increase of 4% from the number of voters in 2019.
The number of voters visiting in 2021 was 299436.
Calculate the 3-year moving average of the number of voters for this period.
Answer:
Number of voters in 2019 = 438048/ 1.04 = 421,200
Therefore, the three year moving average = (421200 + 438048 + 299436)/ 3 = 386,228
Week 197 (7 May 2021)
R2-D2 and Obi-Wan Kenobi set off from the same point at the same time, to travel the same 140 mile journey across Naboo.
Obi-Wan Kenobi travels at 45mph and R2-D2 travels at 35mph.
What will the difference be in their arrival times?
Answer:
Obi-Wan Kenobi would take 140/45 = 3.1111 hours
R2-D2 would take 140/35 = 4 hours
4 – 3.11111 = 0.8888889
0.8888889 x 60 = 53.33334 minutes
0.33333 x 60 = 20 seconds
53 minutes and 20 seconds
Week 196 (30 April 21)
Lucy decides to run 20 miles to train for a marathon. She ran the first half at 5 miles per hour, and the second half at 10 miles per hour. What was her average speed?
Answer:
Time = distance/ speed
First 10 miles = 10/5 = 2 hours
Second 10 miles = 10/10 = 1 hour
So, total time = 2 hours + 1 hour = 3 hours
Av = 20/3 = 6.66 miles/hr
Week 195 (23 April 21)
There are 55 people in a supermarket. Some have been vaccinated, some have not. If the ratio of those vaccinated to those unvaccinated is 3:2, how many have not been vaccinated?
Answer:
2/5 x 55 =
22
Week 194 (16 April 21)
It’s Paul’s birthday today.
Exactly 5 years from now Paul’s father, John will be twice as old as Paul.
The current sum of the ages of John and Paul is 86.
How old is Paul today?
Answer:
J+ 5 = 2(P +5)
J+ P = 86 ; J = 86 – P
Substituting back into the first equation:
86 – P+ 5 = 2 (P + 5)
91 –10 = 3P
3P = 81
P= 27
Week 193 (9 April 21)
A population of rabbits is increasing.
Assume that, each April, the number of rabbits is 4.6% more than it was the previous April.
In April 2005, there were 150 rabbits.
How many rabbits will there be in April 2021?
Answer:
(1.046) ^16 x 150 = 308 rabbits
Alternative answer:
As two people pointed out, technically you can’t have a part of a rabbit reproducing and so we also accepted 296 as a valid answer (but this wouldn’t have got you any marks in the GCSE question!):
| Apr-2005 | 150 | 150 |
| Apr-2006 | 156.9 | 156 |
| Apr-2007 | 164.1174 | 163 |
| Apr-2008 | 171.6668004 | 170 |
| Apr-2009 | 179.5634732 | 177 |
| Apr-2010 | 187.823393 | 185 |
| Apr-2011 | 196.4632691 | 193 |
| Apr-2012 | 205.5005794 | 201 |
| Apr-2013 | 214.9536061 | 210 |
| Apr-2014 | 224.841472 | 219 |
| Apr-2015 | 235.1841797 | 229 |
| Apr-2016 | 246.002652 | 239 |
| Apr-2017 | 257.3187739 | 249 |
| Apr-2018 | 269.1554375 | 260 |
| Apr-2019 | 281.5365877 | 271 |
| Apr-2020 | 294.4872707 | 283 |
| Apr-2021 | 308.0336852 | 296 |
Week 192 (2 April 21)
Amy is organising an Easter egg hunt and buys 5 large eggs and 4 small eggs which cost £4.53. Later that day she buys a further 3 large eggs and 6 small eggs which cost £4.95.
How much would it cost for Amy to buy 1 large egg and 1 small egg in pence?
Answer
5L + 4S = 4.53 Therefore 4S = 4.53 – 5L; S = (4.53 -5L)/4
3L + 6 S = 4.95
3L + 6 x (4.53 -5L)/ 4 = 4.95
12L + 27.18 – 30L = 19.8
7.38 = 18L
L= 0.41
2.05 + 4S = 4.53
4S = 2.48
S= 0.62
L+ S = 0.41 + 0.62 = 103p
PS if you’re wondering why the small eggs are more expensive they were filled with fondant while the large eggs were hollow
Week 191 (26 March 21)
The angles of elevation of the top of a tower from two points A and B are 26º and 38º respectively.
If AB is 10m, find the height of the tower.

Answer:
Angle ACB = 12 (38 -26)
BC/ sin26 = 10/ sin12
Therefore BC = 21.08
Sin38 = CD / BC
Therefore CD = 12.98 m
Week 190 (19 March 21)
An online business can place 8 large boxes or 10 small boxes into a carton for shipping. In one shipment, they sent a total of 96 boxes. If there are more large boxes than small boxes and they send both sizes of boxes, how many cartons did they ship?
Answer:
The number of large boxes must be 16 or 56 or 96 (to give a “6” at the end of the number)
So the number of small boxes would be 80 (96 –16) or 40 (96 – 56) or 0 (96-96)
However it can’t be 80 small boxes (as this would be more than the 16 large boxes) and it can’t be 0 as they send some small boxes), so they must send:
56 large and 40 small, which means they send 11 cartons (7 + 4)
Week 189 (12 March 21)
Whilst waiting in the green room, Meghan asks Harry to remove two cards from a pack of 52 cards. What is the probability that Harry selects two cards of the same suit?
Answer:
The first card can be from any suit. Therefore, you just consider the second card which would be:
12/51
= 4/17
Week 188 (5 March 21)
As part of his home-schooling responsibilities, Rishi asks his children what is the lowest positive number (other than 1) that is not prime nor the sum of two primes?
Can you help him solve the problem?
Answer:
Non-prime numbers (ignoring 1):
4 = 2+2
6 = 3+3
8 = 3+5
9 = 7+2
10 = 5+5 or 7+3
12 = 5+7
14 = 11+3
15 = 13+2
16 = 13+3
18 = 13+5
20 = 17+3
21 = 19+2
22 = 19+3
24 = 19+5
25 = 23+2
26 = 23+3
27
Week 187 (26 February 21)
30 people were asked if they prefer to go on holiday in England or in Scotland or in Wales
19 of the people were male.
5 of the 16 people who said England were female.
4 males said Scotland
6 people said Wales
One of the females is chosen at random
What is the probability that this female said Wales?
Answer:
| England | Scotland | Wales | Female/Male |
| 5 | 4 | 2 | F (11) |
| 11 | 4 | 6 | M (19) |
Week 186 (19 February 21)
On 13th February 1971 Pat went into a shop with £1 and a florins in her purse. She spent half a crown on sweets. How much did she have left in old currency (pounds, shillings and pence)?(click on the answer button for a clue)
Answer:
So you need to know:
1 shilling = 12 (old) pence
20 shillings = 1 £ (old) pound
1 crown = 5 x shillings
1 florin = 2 x shillings
£1 pound = 19 shillings and 12 pence
1 florin = 2 shillings
Half a crown = 2 shillings and 6 pence
Therefore Pat would have left the shop with:
19 shillings and 6 pence
Week 185 (12 February 21)
Paul owns a clothing store in London. He has created a unique way of pricing items. A jumper costs £30, a coat cost £20, a hat costs £15 and a scarf costs £25. Using his method, how much would a cardigan cost?
Answer
Each item is priced by multiplying the number of letters in the word by 5, so cardigan has 8 letters:
8 x 5 = £40
Week 184 (5 February 21)
Rebecca needs a new circular kitchen table with a diameter of 120cm. She knows the cost of a circular table is directly proportional to the square of the radius. A circular table with a radius of 40cm costs £50. How much will Rebecca’s new table cost?
Answer:
C = k r2
Find k:
50 = k 40 x 40
k = 50/ 1600 = 1/ 32
Radius of new table = 120/ 2 = 60
So:
C = 1/32 x 60 x 60 = 112.50
Week 183 (29 January 21)
Triangle ABC is such that:
AB = 9cm BC = 12cm and AC = 7cm. Find angle BAC
(Clue – you’ll need the cosine rule!)
Answer
Cos A = (b2 + c2 – a2)/2bc
Cos A = (81 + 49 – 144)/126
A = 96.38 degrees
Week 182 (22 January 21)
There are some counters in a bag.
The counters are red or white or blue or yellow.
James is going to take at random a counter from the bag.
The probability that the counter will be blue is 0.45 and that it will be yellow is 0.25.
There are 18 blue counters in the bag.
The probability that the counter James takes will be red is twice the probability that the counter will be white.
Work out the number of red counters in the bag.
Answer:
Prob Red + Prob White + Prob Blue + Prob Yellow = 1
Therefore:
P(R) + P(W) + 0.45 + 0.25 = 1
P(R) = 2 x P(W)
Therefore:
P(R) + P(R)/ 2 = 1 – 0.7
P(R) = (0.3 x 2 )/ 3 = 0.2
Number red = 0.2/0.45 x 18 = 8
8 red counters
Week 181 (15 January 21)
A goods train normally travels 60 miles at a certain speed. One day, due to bad weather, the train’s speed is reduced by 10m.p.h., so that the journey takes 3 hours longer. Find the normal speed.
Answer:
D = S x T
60 = S x T; T = 60/S
60 = (S – 10) x (T + 3)
Substituting in:
60 = (S -10) x (60/S +3)
60 = 60 + 3S – 600/S -30
30 = 3S -600/S
30S = 3S2 – 600
3S2 – 600 -30S = 0
S2 -10S – 200 = 0
(S + 10) (S – 20) = 0
S = 20 (-10 not possible)
Therefore 20 mph
Week 180 (8 Jan 20)
How many triangles in the picture below?

Answer:
18
6 in the top row
6 in 2nd row
6 in bottom row
Week 179 (25 Dec 20)
Ellie the Elf has been naughty! She has painted numbers on to Christmas tree baubles in the following order:
2, 3, 5, 9, 18, 34, 66, 131…
Can you work out what number has been painted on the last bauble?
Answer:
Firstly, write out the exponential sequence of 2:
1, 2, 4, 8, 16, 32, 64, 128, 256
Then write out the number of digits in each number in this sequence:
1, 1, 1, 1, 2, 2, 2, 3, 3
Then add the two sequences together!!
So the next number would be 256 + 3 = 259
Simples (especially without the sherry 
Week 178 (18 Dec 20)
Taking the population of England to be 56 million of which 15% are over 70.
It is hoped that, from today, 80% of the over 70s will have the Covid 19 vaccination, however only 50% of those aged 70 and under are expected to have it.
If each vaccination takes 5 minutes to administer by a clinician and 2 vaccinations are needed per person, how many clinicians are needed to complete all vaccinations in the next year (assuming that each clinician works 8 hours a day, 5 days a week, 46.4 weeks a year).
Answer:
Over 70s having injection = 56m x 15% x 80% = 6,720,000
Under 70s having injection = 56m x 85% x 50% = 23,800,000
Total = 30.52m
Hours required = 30.52m x 2 x 5/60 = 5,086,667 hours
Hours per clinician = 8 x 5 x 46.4 = 1,856 hours
Number of clinicians = 5,086,667/ 1856 = 2740.66 = 2,741 clinicians
Week 177 (11 Dec 20)
Carolyn was in the pet shop buying bird food. One bag had a height of 3 cm and cost £1.10. Another mathematically similar bag has a height of 6 cm. and costs £4.00. If the bird food had been sold according to its weight how much will Carolyn save by buying the larger bag at the reduced cost?
Answer:
Scale factor = 2
Hence:
Volume factor = 2^3 = 8
Weight of large bag is 8 times weight of small bag
i.e. cost of large bag = £8.80
So saving is £4.40 (8.80 – 4)
Week 176 (4 Dec 20)
In the Debenhams closing down sale, they have coats being sold at a price of £85.05. This is a reduction in the original sales price of 55%. If the profit margin was originally 40%, how much did Debenhams pay for each coat?
Answer:
Let P = original price of coat
Then 0.45 * P = 85.05
P = 189
C = 0.60 * 189 = £113.40 (not £113.14 as stated in the email!)
Week 175 (27 Nov 20)
Bethan is waiting at the hairdressers for a long overdue hair cut. She opens up the newspaper and in the quiz section notices the following series to solve:
3 9 72 18 342 927 7812 1656 ?
Can you help Bethan work out what number comes next?
Answer:
38691
(each number is x 3 and then mirrored/ reflected in y axis)
Week 174 (20 Nov 20)
A sheet of paper is 8 x 10-3 cm thick.
Tom wants to place 400 sheets of paper into the tray of his home printer. The tray is 3cm deep.
Is the tray deep enough for 400 sheets of paper (please explain your answer)?
Answer:
400 x 8 x 10-3 = 4 x 102 x 8 x 10-3 = 32 x 10-1
= 3.2 cm
As the tray is only 3cm deep the 400 sheets won’t be able to fit
0.2 = Y x 8 x 10-3
2 x 10-1/ 8 x 10-3 = 2 x 102 / 8 = 200/8 = 25 sheets left over
Week 173 (13 Nov 20)
Jesse has a jar of sweets.
There are 414 red, 39 blue, 240 violet and 77 orange sweets.
What chance does Jesse have of picking an orange one with his eyes shut?
Answer:
77/770 = 10% or 1/10
Week 172 (6 Nov 20)
Joe Biden is getting ready for his victory speech and is about to put on some socks. The sock drawer contains 10 Stars socks and 10 Stripes socks. How many socks will Joe need to pull out a sock drawer to ensure that he has a matching pair (of either the Stars or the Stripes)?
Answer:
With three socks there is always a matching pair since. Either you will have chosen three of the same style, or a matching pair and an odd one out.
Week 171 (30 October 20)
Justine buys a new car for £25000.
The value of this car will depreciate by 20% at the end of the first year
and then by 12% at the end of every year after the first year (based on the value at the start of the year).
What is the value of the car at the end of 3 years?
Answer:
End of first year = 0.8 X 25000 = 20000
End of year 2 = 0.88 x 20000 = 17,600
And then at the end of year 3 = 0.88 x 17600 = 15488
Week 170 (23 October 20)
On Monday 4 bricklayers took 3 hours to lay a total of 4200 bricks
On Tuesday there are only 2 bricklayers.
How many hours will it take the 2 bricklayers (assuming that they work at the same rate as the workers on Monday) to lay a total of 3150 bricks?
Answer:
12 man hours for 4200
Therefore 350 bricks per man hour
3150/350 = 9 man hours, so would take 2 people 4.5hours
Week 169 (16 October 20)
Ian is in his factory with a solid metal cuboid in his hands. This cuboid has three faces with areas of 27 cm^2, 15 cm^2 and 45 cm^2.
The lengths, in cm, of the edges of the cuboid are whole numbers.
Ian decides that in the current economic climate it would be better to turn the cuboids into smaller cubes by melting it.
Each of the cubes has sides of length 2.5 cm.
What is the greatest number of these cubes that can be made?
Answer:
27 = 3 x 9
15 = 3 x 5
45 = 5 x 9
Therefore the sides are 3, 5 and 9 and so the volume of the cuboid is 135 cm^2
Each new cube has a volume of 15.625 cm^2
Therefore 135/ 15.625 = 8.64
So there would be 8 new cubes
Week 168 (9 October 20)
Steph saw her favourite packet of brazil nuts being sold as “Buy one get one half price”. However just for today we are giving an extra 20% off the final price.
Steph buys two packets, what percentage does Steph save overall?
Answer:
Cost = (x + 0.5x) x 0.8 = 0.8x + 0.4x = 1.2x
Saving = 2x – 1.2x = 0.8x
% saving = 0.8/ 2 * 100% = 40%
Week 167 (2 October 20)
Christopher is going to paint a floor in the shape of a trapezium with parallel sides of lengths 10 m and 16 m. The perpendicular height between the parallel sides is of length 7 m.
Answer:
Each 5 litre tin of paint costs £16.90.
1 litre of paint covers 2 square metres.
How much will he need to spend on buying paint?
Answer:
Area = (10 + 16) / 2 x 7 = 91 m^2
91/2 = 45.5
Therefore he will need to buy 10 tins of paint (ie 5 x 10 = 50 litres)
So cost = 16.90 X 10 = £169
Week 166 (25 September 20)
During Paula’s tour of New Zealand last year she caught a plane and flew from Auckland to Gisborne on a bearing of 115 degrees.
The plane then flew on to Wellington on a bearing of 232 degrees.
The distance from Wellington to Gisborne is 400 km.
The distance from Auckland to Wellington is 410 km.
Calculate the bearing of Wellington from Auckland.
(You need to use The Sine Rule – good revision for anyone with children in year 11!)
Answer:
Drawing the flight you would end up with a triangle A G W, where the length AW = 410 and the length WG = 400
The angle WGA = 63, so using the sign rule:
sin(WAG) / 400 = sin 63/ 410
Therefore Sin(WAG) = 0.8693
So Angle WAG = 60.37
So bearing = angle WAG + bearing from A to G = 60.37 + 115 = 175.37 degrees (to 2dp)
Week 165 (18 September 20)
Nicola and Rob have gone out for their anniversary. In the restaurant there are:
– 9 starter dishes
– 15 main dishes
– 8 dessert dishes
Nicola is going to choose one of the following combinations for her meal.
-a starter dish and a main dish
or
-a main dish and a dessert dish
or
-a starter dish, a main dish and a dessert dish.
How many different ways are there for Nicola to choose her meal?
Answer:
9 x 15 + 15 x 8 + 9 x 15 x 8 = 1,335 (that’s a lot of combinations!)
Week 164 (11 September 20)
This morning it took Natalie 10 minutes to do the school run in her car. For the first half of the distance the speed was 60 km/hr and for the second half of the distance the speed was 40 km/hr. How far is the journey?
Answer
Kate’s mum’s method (the quick way to do it):
Let total distance travelled be x km
Then using Time = Distance / Speed
x / (2×60) + x /(2×40) = 10/60
Multiply by 240
2x +3x = 40
x = 8km
Kate’s method (not quite as efficient!)
D = S x T
D1 = 60 x T1
D2 = 40 x T2
T1 + T2 = 10/60
T1 = 10/60 – T2
Substituting in:
D1 = 60 X (10/60 – T2) = 10 – 60T2
As D1 = D2
10 – 60T2 = 40T2
100T2 = 10
Therefore T2 = 10/100
So D2 = 40 x 10/100 = 4
Therefore total distance = 8km (ie 2 x D2) – she took 4 minutes to travel the first 4km and 6 mins to travel the second 4 km.
Week 163 (4 September 20)
Kate’s dog has just given birth to seven puppies. The mean birth weight of the last six puppies was 370 g, but the mean birth weight of all seven puppies was 382 g. What was the weight of the first puppy to be born?
Answer:
Total weight of 7 puppies = 7 x 382 = 2674 g
Subtract:
Total weight of 6 puppies = 6 x 370 = 2220 g
Weight of 7th puppy = 454 g
Week 162 (28 August 20)
Complete this sequence
02 80 82 59 18 43 74 66 47 34 81 95 ?? ?? ??
Answer:
It is a palindrome sequence with symmetry around number 66, so the numbers are:
28 08 20 (which happens to be the date the quiz was set!)
Week 161 (21 August 20)
In 3 years’ time Willow the dog will be as old as her owner was 20 years ago. Their present ages total 51 years. Find the age of each now.
Let W = Willow’s age now and X = Owner’s age now
W + 3 = X – 20; W = X – 23
W + X = 51; W = 51 – X
Therefore:
51 – X = X -23
2X = 51 + 23
X = 74/2 = 37 years
W = 14 years
Therefore Willow is 14 and her owner is 37.
Week 160 (14 August 20)
It is a Wednesday evening during August and Rob and Barbara have taken their three children to a restaurant operating the Eat Out to Help Out scheme where you get 50% off food and non alcoholic drink on Monday, Tuesday and Wednesday in August, to a maximum of £10 each.
Before deductions their bill was :
| Food | Drink | |
| Rob | £22.70 | £8 wine |
| Barbara | £16.50 | £8 wine |
| Jack | £12.40 | £5 non-alcoholic |
| Lucy | £13.80 | £7 non-alcoholic |
| Megan | £11.20 | £4 non-alcoholic |
How much did they actually pay?
Answer
As we are not expecting you to know the finer details of the government scheme (which apparently averages the discount out over the number of people) we are accepting two answers to this question:
- Discount applied strictly on an individual basis:
| Food | Drink | Discount | |
| Rob | £22.70 | £8 wine | (22.7)/2 = 11.35, capped at 10 |
| Barbara | £16.50 | £8 wine | (16.5)/2 = 8.25 |
| Jack | £12.40 | £5 non-alcoholic | (12.4+5)/2 = 8.7 |
| Lucy | £13.80 | £7 non-alcoholic | = (13.8 + 7)/2 = 10.40, capped at 10 |
| Megan | £11.20 | £4 non-alcoholic | 15.2/2 = 7.6 |
| £76.60 | £32 | Total discount = 44.55 |
Therefore bill = 108.6 – 44.55 = 64.05
2. If the discount is averaged out over the 5 people, then the discount would be:
(76.6 + 5 + 7 + 4)/ 2 = 46.30
Therefore the bill would be = 108.6 – 46.30 = 62.30
Week 159 (7 August 20)
While Kate was on holiday in Scotland, she walked 10 kilometres to a waterfall at an average speed of x kilometres per hour.
She returned from the waterfall in a hurry as a big rain cloud was looming and so this time she walked at an average speed of (x +1) k.p.h..The time of the return journey was 30 minutes less than the time of the first journey.
Find the time Kate took to walk to the waterfall.
Answer:
T = D/S
On the way out:
T = 10/x , so x = 10/T
On the way back:
T – 0.5 = 10/(x + 1)
Therefore:
T – 0.5 = 10/ (10/T +1)
T( 10/T +1) – 0.5( 10/T +1) = 10
20 + 2T – 10/T – 1 = 20
2T^2 – 10 – T = 0
(2T – 5)(T + 2) = 0
T = -2 not poss
Therefore:
2T = 5, T = 2.5 hrs
Week 158 (31 July 20)
As I was coming from St Ives I met a man with x wives, each wife had y sacks, each sack had x cats, each cat had x kits, each kit had z balls. Balls, kits, cats, sacks, wives and the man: 700 were going to St Ives. What were x y and z ?
1 + x3yz + x3y + x2y + xy + x = 700
Therefore
x3yz + x3y + x2y + xy + x = 699
x [ x2yz + x2y + xy + y + 1] = 699
The only factors of 699 are 699, 1, 3 and 233 (233 is a prime number)
The question implies that he has more than one wife, so x must be 3 and therefore:
x2yz + x2y + xy + y + 1 = 233
So:
9yz + 9y + 3y + y = 232
9zy + 13y = 232
y (9z + 13) = 232
The factors of 232 are 1, 232, 2, 116, 4, 58, 8, 29
Again as the question implies there is more than one sack, y could be 2, 4 or 8.
If y = 2
9z + 13 = 116, therefore 9z = 103 (z is not an integer)
If y = 4
9z + 13 = 58, therefore z = 5
If y = 8
9z + 13 = 29, therefore 9z = 16 (z is not an integer)
So x = 3, y = 4, z = 5
Week 157 (24 July 20)
Abigail’s Grandfather clock is currently running nine times too fast. At 5:30 its hands were pointing to the right time. When did it next tell the right time?
Answer:
Let them be at the same time after T hours.
T + 12x = 9T
8T = 12x
T = 1.5x
Therefore, when x = 1, T = 1.5, so the next time would be 5.30 + 1.5 hours = 7:00
Week 156 (17 July 20)
What is the area of the smallest square which can contain a circle which can contain a square which can contain a circle with area π cm2
Answer:
Area of the circle = π r2
So:
π = π r2
Therefore r =1
So if the smallest circle has radius of 1, it has a diameter of 2 and so the smallest square has sides of 2 cm.
The diagonal of that square is the diameter of the largest circle which also happens to be the length of the sides of the largest square and can be found by using the formula:
a2 + b2 = c2
As both a and b are the same (because it’s a square):
2a2 = c2
And we know a = 2, therefore
c = 2 √ 2
So, as this is the length of the side of the largest square, it’s area will be:
Area = (2 √ 2) x (2 √ 2) = 4 x 2 = 8 cm2
Week 155 (10 July 20)
At a pre-Covid 19 party, everyone shook hands with everybody else. There were 66 handshakes. How many people were at the party?
Answer
In general, with n+1 people, the number of handshakes is the sum of the first n consecutive numbers: 1+2+3+ … + n.
Since this sum is n(n+1)/2, we need to solve the equation n(n+1)/2 = 66.
This is the quadratic equation n^2+ n -132 = 0.
(n-11)(n+12) = 0
Therefore n = 11 or -12
As -12 is not possible, n has to be 11 and so n+ 1 = 12. Therefore there are 12 people at the party.
Week 154 (3 July 20)
When cars go round a bend there is a force, F between the tyres and the ground.
F varies directly as the square of the speed, v .
When v = 40, F = 18
Find F when v = 32.
Answer:
Let F = K v2
Therefore we need to find K first and substitute in:
18 = 1600 K
Therefore K = = 18/1600 = 9/800
So when v = 32
F = 9/ 800 x 1024
= 1

